This thesis investigates theoretical and practical aspects of conformal geometry in ideal magnetohydrodynamics. The main results relate stationary points of a hierarchy of L2 resp. L1-optimization problems, which are distinguished by the topological constraints they impose, by a conformal change of metric. In particular, they establish a conformal equivalence between force-free fields and geodesic vector fields as well as harmonic fields and eikonal fields.
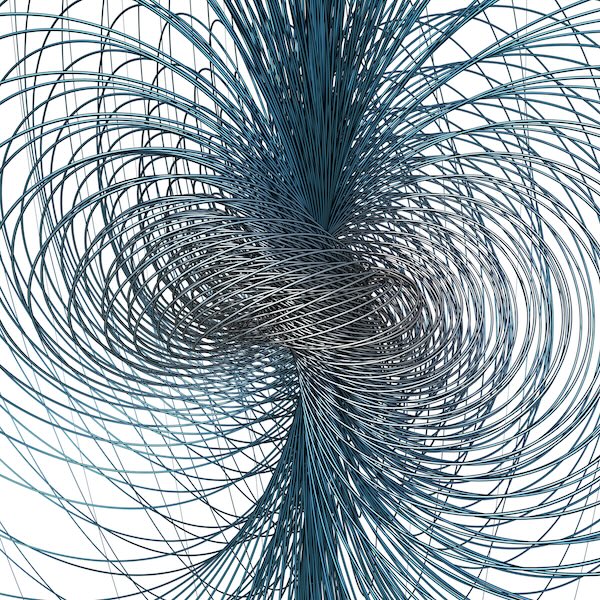
Based on a geometric and structure preserving discretization of ideal plasma, so-called magnetic relaxation is interpreted from the viewpoint of conformal geometry, which gives rise to a novel Lagrangian model based on discrete plasma filaments which is less geometrically rigid than previous approaches. The practical applicability of the proposed model is demonstrated by two examples: First, we approximate stationary states of knotted or linked plasma filaments. Second, the presented model is part of a procedural pipeline that generates stellar atmospheres for computer graphics purposes.
|