|
|
|
|
|
|
|
|
|
Abstract |
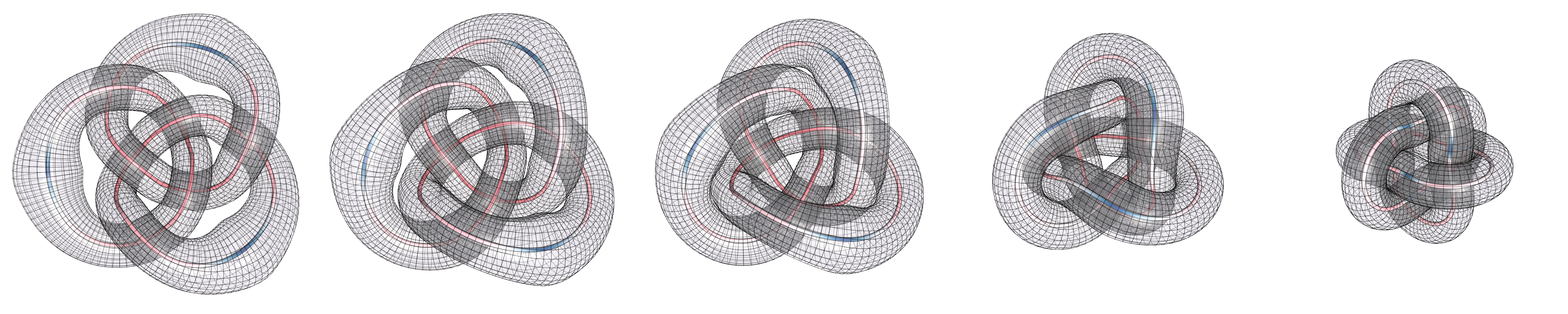
| We present a Lagrangian method for the computation of ideal plasma knots and links. It is based on a variational principle for stable equilibria of an ideal plasma in the case of a free boundary subjected to external magnetic or plasma pressure forces. For this purpose, we introduce a structure preserving discretization of plasma based on decompositions of Riemannian manifolds representing pressure confined plasma regions in magnetohydrostatic equilibrium. Moreover, we show that, by the virtue of an analogy, the method can be used for the approximation of steady Euler-flows of arbitrarily complex topology. |
Resources |
|
 |
Plasma Knots Oliver Gross, Ulrich Pinkall and Peter Schröder Physics Letters A 480 (2023) 128986, https://doi.org/10.1016/j.physleta.2023.128986 [Preprint] |
Acknowledgement |
| This work was funded by the Deutsche Forschungsgemeinschaft (DFG - German Research Foundation) - Project-ID 195170736 - TRR109 "Discretization in Ge- ometry and Dynamics", the Caltech Center for Informa- tion Science & Technology, and the Einstein Foundation Berlin. Additional support was provided by SideFX software. |